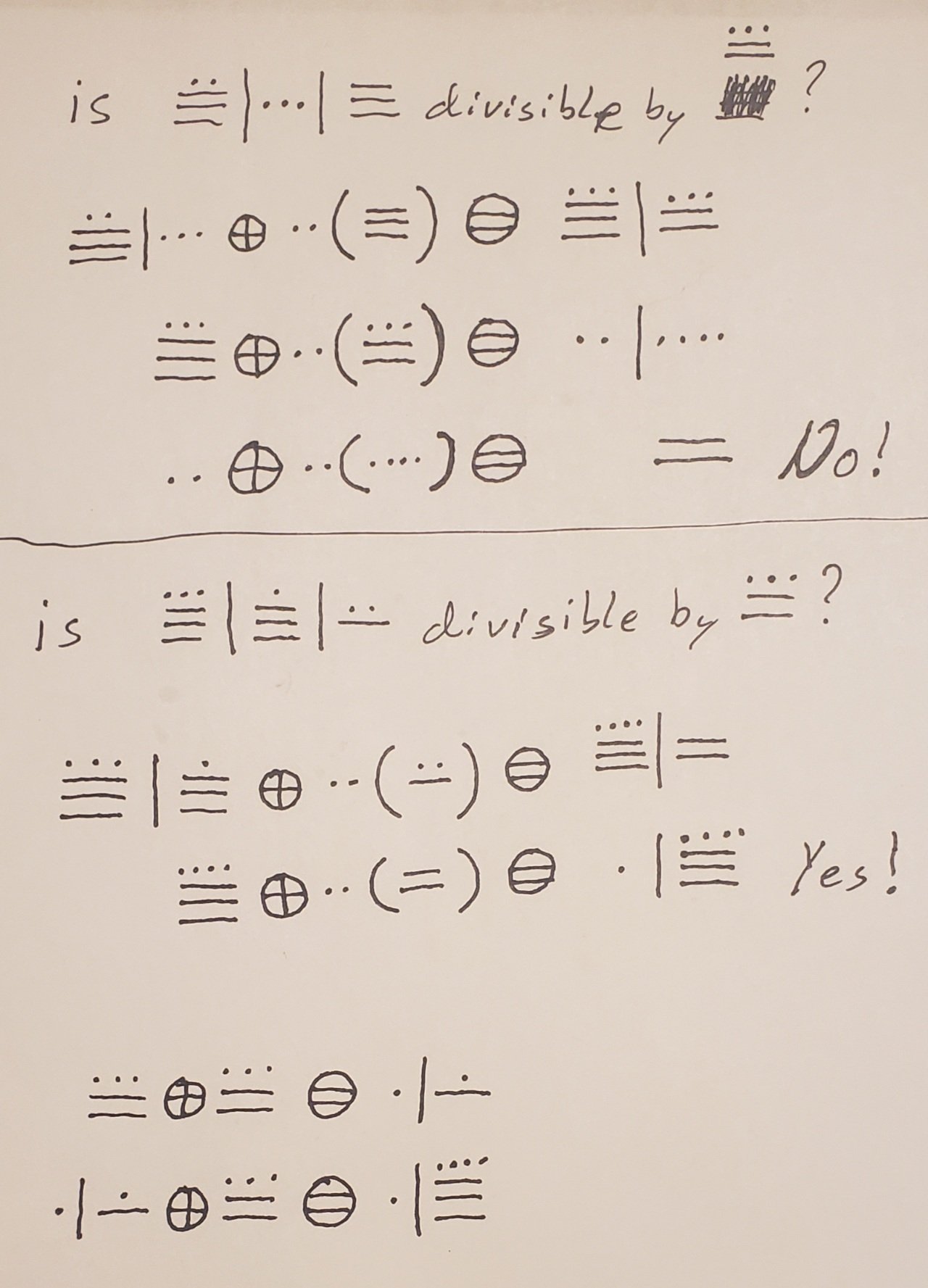Since I have studied mesoamerican languages, which includes base 20 numbering systems, I was curious to see what may happen by following the same rules for 7 in decimal.
The following will be written as follows.
7*x : 5
Which implies any number that is divisible by 7 can be found by the following programmable formula (ignoring integer division) (y - y % 10) / 10 + (y % 10) * 5 where y = 7 * x (Erm, just watch this video).
Maya Number : Multiplier
7*x : 6
13*x : 2
17*x : 6
Just what I've seen so far...Am needing sleep, and am older and a lot more decrepit, so have no clue if I'll ever work this out fully and completely in the future, heh. Meh, it was fun while it lasted...Time for sleep...
If anyone else wants to play around with it, I do have a few pari/gp functions written that can be used to work with it.
\\ Maya(x) convert a decimal number x into a Mayan number as a list.
Maya(x) = { V=List();while(x>0,listinsert(V,x%20,1);x=x\20);return(V) }
\\ Maya2Dec(X) convert a Maya number list X to a decimal number.
Maya2Dec(X) = { t=0;for(x=1,length(X),t=t*20+X[x]);return(t) }
g(x,t) = x\20+(x%20)*t;
Edit:
Since this was rather confusing, I'll try a different method to explain this.
A base 20 system, such as the Maya or Nahuatl/Aztecs counted in, the single digits would be 0,1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19, as they counted with both fingers and toes.
Another way to aid in this, in the Tzotzil Maya language, they count based on a person or persons.
20 = jtob
21 = jun scha'vinik (2nd person's one [digit])
22 = chibal scha'vinik (2nd person's two [digits])
39 = balunlajunebal scha'vinik (2nd person's 19 [digits])
40 = cha'vinik ([the whole] 2nd person. Each person has 20 digits, so including the second person is 40 digits all together).
Now, with that, lets look over the 13\x : 2 that I wrote above.
Is the number 6875 divisible by 13? In a base 20, 6875 would be written as 17:3:15, using the colon to differentiate the digits from a base 10 system. Thus, 17 * 20^2 + 3 * 20 + 15 = 6875.
- 17:3 + 2 * 15 = 18:13 (Base 10 ; 343 + 30 = 373)
- 18 + 2 * 13 = 2:4 (Base 10 ; 18 + 26 = 44)
- 2 + 2 * 4 = 10 (No! This is not divisible by 13).
Is the number 7527 divisible by 13?
- 18:16 + 2 * 7 = 19:10 (Base 10 ; 376 + 14 = 390)
- 19 + 2 * 10 = 1:19 (Base 10 ; 19 + 20 = 39. Check, this is divisible by 13).
Hopefully that is better.
Edit 2:
And here is the work in Maya. (Using circles around the + and = signs to make it easier to read. Plus, using very well defined parentheses to differentiate between the line used for the digit spacer and as showing multiplication).