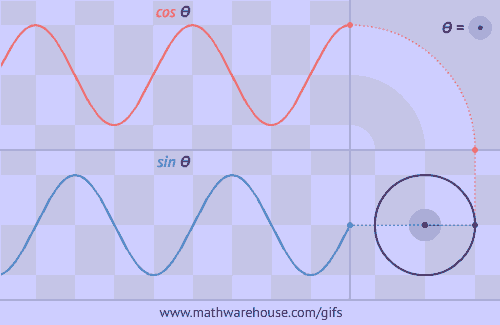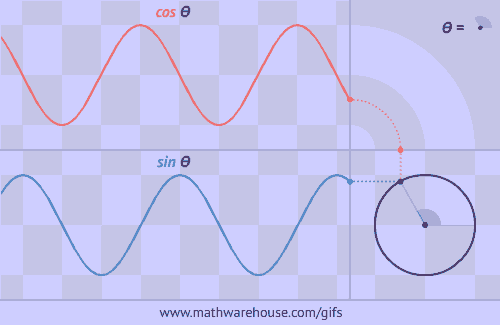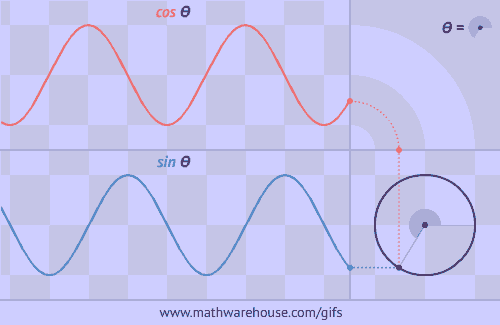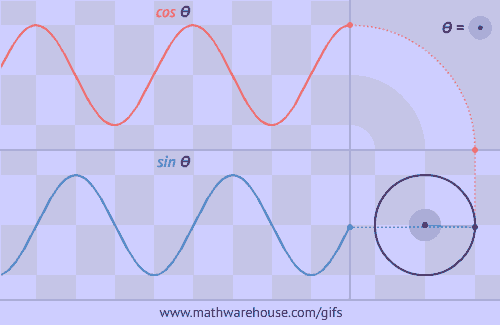
This triangle is impossible.
If the distance between B and C is 0, B and C are the same points. If that is the case, the distances between A and B and A and C must be the same.
However, i ≠ 1.
If you want it to be real (hehe) the triangle should be like this:
C
| \
|i| | \ 0
| \
A---B
|1|
Drawing that on mobile was a pain.
As the other guy said, you cannot have imaginary distances.
Also, you can only use Pythagoras with triangles that have a 90° angle. Nothing in the meme says that there's a 90° angle. As I see it, there are only 0° and 180° angles.
Goodbye, I have to attend other memes to ruin.